The Historical Roots of Elementary Mathematics

Lucas N. H. Bunt, Phillip S. Jones, Jack D. Bedient

• Editore: Dover Publications
• Anno: 2012
• ISBN:
  9780486139685
• Argomento: Mathematics
• Categoria: History & Philosophy of Mathematics

`Will delight a broad spectrum of readers.` — American Mathematical Monthly.
Do long division as the ancient Egyptians did! Solve quadratic equations like the Babylonians! Study geometry just as students did in Euclid's day! This unique text offers students of mathematics an exciting and enjoyable approach to geometry and number systems. Written in a fresh and thoroughly diverting style, the text — while designed chiefly for classroom use — will appeal to anyone curious about mathematical inscriptions on Egyptian papyri, Babylonian cuneiform tablets, and other ancient records.
The authors have produced an illuminated volume that traces the history of mathematics — beginning with the Egyptians and ending with abstract foundations laid at the end of the nineteenth century. By focusing on the actual operations and processes outlined in the text, students become involved in the same problems and situations that once confronted the ancient pioneers of mathematics. The text encourages readers to carry out fundamental algebraic and geometric operations used by the Egyptians and Babylonians, to examine the roots of Greek mathematics and philosophy, and to tackle still-famous problems such as squaring the circle and various trisectorizations.
Unique in its detailed discussion of these topics, this book is sure to be welcomed by a broad range of interested readers. The subject matter is suitable for prospective elementary and secondary school teachers, as enrichment material for high school students, and for enlightening the general reader. No specialized or advanced background beyond high school mathematics is required.

1

EGYPTIAN MATHEMATICS

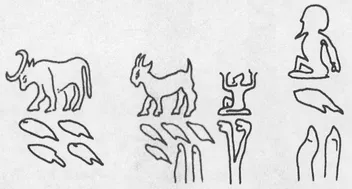
1-1 PREHISTORIC MATHEMATICS

The earliest written mathematics in existence today is engraved on the stone head of the ceremonial mace of the Egyptian king Menes, the founder of the first Pharaonic dynasty. He lived in about 3000 B.C. The hieroglyphics on the mace record the result of some of Menes’ conquests. The inscriptions record a plunder of 400,000 oxen, 1,422,000 goats, and 120,000 prisoners. These numbers appear in Figure 1-1, together with the pictures of the ox, the goat, and the prisoner with his hands behind his back. Whether Menes exaggerated his conquests is interesting historically but does not matter mathematically. The point is that even at this early date, man was recording very large numbers. This suggests that some mathematics was used in the centuries before 3000 B.C., that is, before the invention of writing (the prehistoric period).

Figure 1-1 From a drawing (Plate 26B) in J. E. Quibell, Hierakonopolis, London, 1900.

There are two ways of learning about the mind and culture of prehistoric humans. We have learned about them through the discovery of ancient artifacts, which were found and interpreted by archaeologists. We have also learned about prehistoric civilization by observing primitive cultures in the modern world and by making inferences as to how prehistoric thought and customs developed. In our study of the development of ideas and understandings, both approaches are useful.

One of the most exciting archaeological discoveries was reported in 1937 by Karl Absolom as a result of excavations in central Czechoslovakia. Absolom found a prehistoric wolf bone dating back 30,000 years. Several views are shown in Figure 1-2. Fifty-five notches, in groups of five, are cut into the bone. The first 25 are separated from the remaining notches by one of double length. Although we do not know how this bone was notched, the most plausible explanation is that some prehistoric man deliberately cut it. Perhaps he was recording the number of a collection, possibly of skins, of relatives, or of days since an event. It is reasonable to assume that he made a notch for each object in the collection that he was counting. If this interpretation is correct, then we can recognize in this prehistoric record rudimentary versions of two important mathematical concepts. One is the idea of a one-to-one correspondence between the elements of two different sets of objects, in this case between the set of notches on the bone and the set of whatever the prehistoric man was counting. The other is the idea of a base for a system of numeration. The arrangement of the notches in groups of 5 and of 25 indicates a rudimentary understanding of a base 5 system of numeration.

Figure 1-2 Prehistoric Wolf Bone. (From London Illustrated News, October 2, 1937.)

Anthropological studies reinforce our belief in the existence of prehistoric number ideas. A study of the western tribes of the Torres Straits, reported by A. C. Haddon in 1889, describes a tribe that had no written language which counted as follows: 1, urapun; 2, okosa; 3, okosa-urapun; 4, okosa-okosa; 5, okosa-okosa-urapun; 6, okosa-okosa-okosa . Everything greater than 6 they called ras. A student of modern mathematics would recognize in this system of counting the beginnings of a base 2 numeration system. If a Torres Strait native had recognized this idea, however, he would have used a different word for 4 and would not have said ras for numbers greater...