Doing Simple Math in Your Head

W.J. Howard

• Editore: Chicago Review Press
• Anno: 2001
• ISBN:
  9781569769874
• Edizione: 1
• Argomento: Mathematics
• Categoria: Mathematics General

Almost all adults suffer a little math anxiety, especially when it comes to everyday problems they think they should be able to figure out in their heads. Want to figure the six percent sales tax on a $34.50 item? A 15 percent tip for a $13.75 check? The carpeting needed for a 12½-by-17-foot room? No one learns how to do these mental calculations in school, where the emphasis is on paper-and-pencil techniques. With no math background required and no long list of rules to memorize, this book teaches average adults how to simplify their math problems, provides ample real-life practice problems and solutions, and gives grown-ups the necessary background in basic arithmetic to handle everyday problems quickly.

1. MAKING THINGS EASIER

You can simplify problems in various ways:…

Numbers are not rigid, static things; they’re malleable and fluid, and can be shaped to meet your needs. A problem comes up involving the number 15. You don’t have to work with it as such. If it suits you, you can think of it as 10 + 5, or 5 × 3, or 30/2—whatever fits your need. How do you know what will fit? You don’t, without a little mental experimenting. And in experimenting a guiding light is the magic number 10, as you’ll see.

A wide variety of problems will succumb to just a few easy-to-use methods: reordering numbers, rearranging them, breaking them up, using equivalents and identities, and approximating and rounding off. Always, the focus is on the number 10; it and its multiples are the easiest numbers to handle. As you review these methods, don’t try to memorize; understand the logic…and then practice. When ready, turn to Chapter 2 for practice, or to Chapter 3 for help in understanding.

Ways of Simplifying

In this chapter: how to simplify problems by

• reordering numbers

• rearranging numbers

• breaking up numbers

• using equivalents

• using identities

• approximating and rounding off

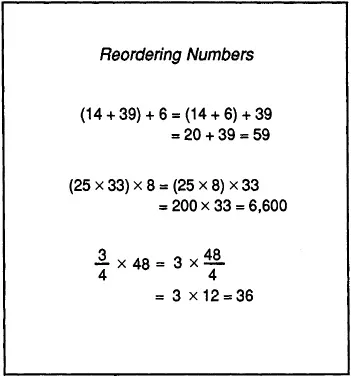
by reordering numbers;…

Numbers come at you helter skelter. You want to add 14, 39 and 6. (Perhaps you’re in a store and thinking of buying some items. In this chapter we won’t worry about why you want to add, multiply, etc.—there will be many examples in the problems later.) The first thing to do is to reorder them to (14 + 6) + 39. Now 14 + 6 gives you 20—a multiple of 10—and 20 is easily added to 39. Result, 59—which you can do in your head in much less time than it takes to tell about it.

You have three numbers to multiply: 25 × 33 × 8. Don’t try to multiply 25 × 33 (this in itself is not too difficult, but you’d still have to multiply by 8); first multiply 8 × 25, getting 200 (aha!, a multiple of 10), and then multiply this by 33. Answer: 6,600.

Maybe a problem involves a fraction: (¾) × 48. You need to multiply 48 by 3 and divide by 4. But by all means, divide first!

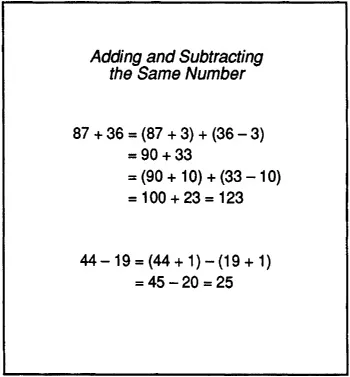
by adding and subtracting a number;…

You want to add 87 and 36. Notice that 87 is close to 90—a multiple of 10. Change 87 to 90 by adding 3, and then, to compensate, subtract that same 3 from 36, getting 33. Rearranging a problem by adding and subtracting the same number doesn’t change the answer. So the original problem of 87 + 36 is now 90 + 33, which is easier. You can make it even easier by adding and subtracting 10: adding 10 to 90 gives 100—a beautiful multiple of 10—and subtracting 10 from 33 gives 23. So the original problem has now been changed to 100 + 23. It doesn’t look anything like the original problem—in fact it’s now no problem at all—but it will give the same answer.

In subtraction, try to change the number being subtracted to a multiple of 10. To subtract 19 from 44, add 1 to 19, giving 20, and add that same number (1) to 44, giving 45. The rearranged problem is now easy: 45 − 20, or 25.

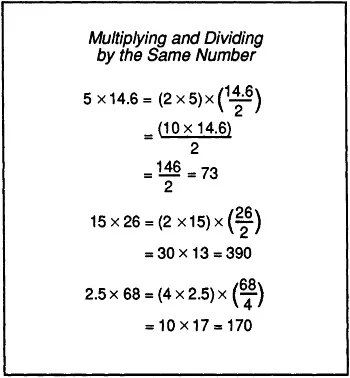
by multiplying and dividing by a number;…

Multiplying or dividing by 2 is easy to do in your head—a fact that has many benefits. To multiply by 5, think of multiplying by 10 and taking half of that. This gives the same result and is often easier. Suppose the problem is 5 × 14.6. Multiplying and dividing by 2 changes it to (2 × 5) × (14.6/2), so we’re now multiplying by 10 (that is, 2x5) and dividing by 2. The result is 146/2, or 73. This also works for numbers ending in 5; multiply by 2 to get a multiple of 10, and divide by 2 to compensate. For example, 15 × 26 is changed to (2 × 15) × (26/2), or 30 × 13, or 390. We divided by 2 before multiplying here, whereas above we did so after—do whatever makes the problem easier.

When you see 2.5 or 25, think of multiplying and dividing by 4, so you’ll be multiplying by 10 or 100 (and dividing by 4). A forbidding-looking 2.5 × 68 is changed to (4 × 2.5) × 68/4, or 10 × 17, or 170. (Also see fraction/decimal equivalents, later.)

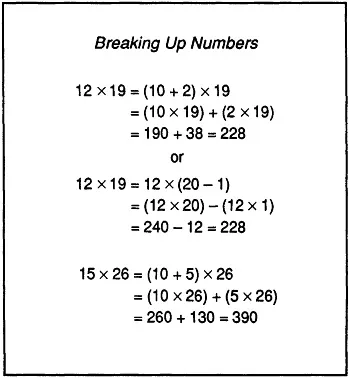
by breaking up numbers…

Many problems give you a choice of how to proceed. Don’t waste time waffling, or you’ll defeat the purpose of making things simple. Consider 12 × 19. Looking for a 10 multiple, you think of 12 as 10 + 2, so the problem is now (10 + 2) × 19, which is (10 × 19) + (2 × 19), or 190 + 38, or 228. (If you have a question about this, see p. 95.) Alternatively, for 12 × 19 you might have thought of 19 as 20 − 1, since 20 is a multiple of 10. The problem then becomes 12 × (20 − 1), or (12 × 20) − (12 × 1), or 240 − 12, or 228 again. If you have trouble with 240 − 12, remember to change the problem so you’re subtracting a multiple of 10. In this case, subtract 2 from each number: 240 − 12 = (240 − 2) − (12 − 2), or 238 − 10, which is 228.

On the previous page we multiplied 15 × 26 by multiplying by 2 and dividing by 2. Instead, we might have broken up 15 into 10 + 5; the problem becomes (10 + 5) × 26, which is 260 + 130, or 390.

in various ways,…

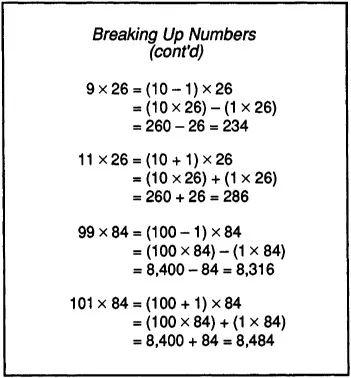
The numbers 9 and 11 are easy to handle, since they’re both so close to 10; think of 9 as 10 − 1 and 11 as 10 + 1. To multiply by 9, first multiply the number by 10 and then subtract the number: 9 × 26 = (10 − 1) × 26 = (10 × 26) − (1 × 26), which is 260 − 26. Some may wish to simplify even further by adding 4 to each number: 260 − 26 = (260 + 4) − (26 + 4), which is 264 − 30, or 234.

To multiply by 11, multiply by 10 and add the number: 11 × 26 = (10 + 1) × 26, which is (10 × 26) + (1 × 26), or 260 + 26, or 286.

You can even handle larger numbers, like 99 and 101, since they’re so close to 100. For example, 99 × 84 = (100 − 1) × 84, or (100 × 84) − (1 × 84), or 8,400 − 84. You can simplify to (8,400 + 16) − (84 + 16), or 8,416 − 100, or 8,316. If the problem is 101 × 84 it’s even easier: 101 × 84 = (100 + 1) × 84, or (100 × 84) + (1 × 84), or 8,400 + 84, or 8,484.

including factoring;…

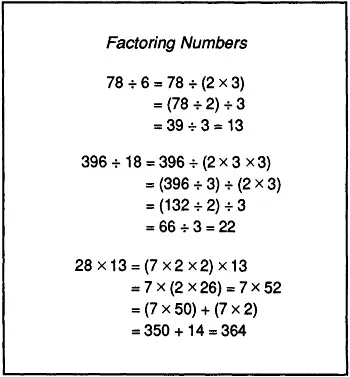
When multiplying or dividing, it’s often useful to factor numbers (composite numbers can be factored into their components: 6 = 2 × 3, for example—see p. 97). Suppose you want to divide 78 by 6. You can perhaps do this directly in your head, but if you have difficulty, divide first by 2 and then by 3. Dividing by 2 gives you 39, and dividing this by 3 gives you the answer, 13.

Another problem: divide 396 by 18. Since 18 = 2 × 3 × 3, you can divide first by 3, getting 132, then by 2, getting 66, then by 3 again, getting the final answer, 22. You might also solve this directly by noting 20 × 18 is 360, and there’s still 2 × 18 to go.

Suppose you want to multiply 28 × 13. Since 28 = 7x2x2, you can restate this problem as (7 × 2 × 2) × 13, which is 7 × (2 × 26), or 7 × 52. For 7 × 52 you can break up 52 into 50 + 2 and multiply separately: (7 × 50) + (7x2) is 350 + 14, or 364.

by using fraction/decimal equivalents…

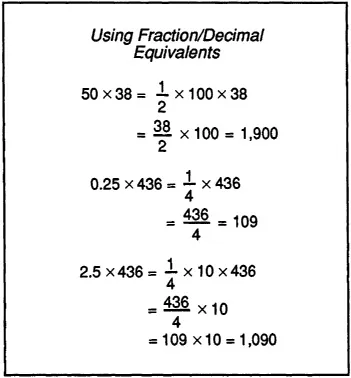
Fractions are surprisingly useful, particularly in multiplication problems (see p. 114). To multiply 50 × 38, it’s easy to multiply 100 × 38 and take half of that—1,900. Another problem: 0.25 × 436. You’d have quite a time multiplying this in your head. But realizing that 0.25 is ¼, you just divide 436 by 4 and have the answer, 109.

Once you become familiar with fractions and their decimal equivalents, you find many opportunities to use them. It’s not just in the form of numbers less than one that fractions are useful. If the second problem above had been 2.5 × 436, you’d do the same thing—divide by 4—but then, since 2.5 is 10 times 0.25, you’d have to multiply by 10 to get the answer: 1,090. With practice, you get in the habit of looking for numbers that correspond to fractional equivalents, knowing you can multiply or divide by a power of 10 (10,100, etc.—see p. 98) to make the answer come out right.

to convert awkward numbers…

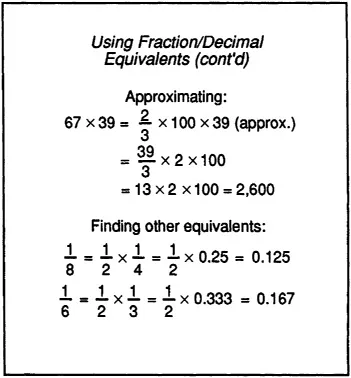
Some fractional equivalents are familiar; it’s common knowledge that ½ = 0.5,¼ = 0.25, and ¾ = 0.75. Also, 1/3 = 0.33 and 2/3 = 0.67 (to two places). A problem involving these numbers can often be handled quickly. For example: 67 × 39 seems at first like a difficult problem. But think of 67 as (2/3) × 100. Note also that 1/3 of 39 is 13, so 2/3 is 26, and you have an approximate answer right away: (2/3) × 100 × 39 is 2,600. The answer is not exact since (2/3) × 100 is not exactly 67.

As for the less familiar equivalents: since you know that ¼ is 0.25, you also know that ⅛, being half of ¼, is 0.125. So if a problem involves 125, you’ll think of dividing by 8 and multiplying by the proper power of 10. By dividing by 2 again, you also know that 1/16 is 0.0625. Or take 1/3, which you know is 0.333; 1/6 is half of that, or 0.167, and 1/12 is half of that, or 0.083. Any or all of these relations can come in handy…if you think of them.

to simpler ones;…

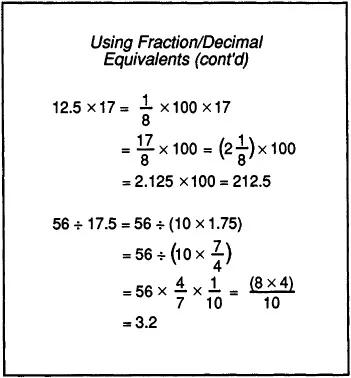
You can do some difficult-looking problems in your head using fraction/decimal equivalents. How about 12.5 × 17? Since ⅛ is 0.125,12.5 is (⅛) × 100, and 12.5 × 17 is (⅛) × 100 × 17. Now 17/8 is 2 ⅛, or 2.125. So 12.5 × 17 is 2.125 × 100, or 212.5. It takes longer to describe it than to do it. Notice that this works even though 8 does not evenly divide 17. Another: 56 ÷ 17.5 looks impossible until you realize 17.5 = 10 × 1.75, and 1.75 = 7/4. So (see p. 106) 56 ÷ 17.5 = 56 × (4/7) × (1/10), which is (8 × 4)/10, or 3.2. Not an easy problem.

If you multiply 200 × 300, you’ll first think “2 × 3 = 6,” then start wondering how many zeros to tack on. This is how to handle a problem using fraction/decimal equivalents. Think about the numbers or digits first, without worrying about size of the answer. This lets you make useful associations. Size is just a matter of factors of 10—how many zeros or where the decimal point goes.

and by using identities…

Try multiplying 5 − 3 by 5 + 3. Yes, the answer will be 2 × 8, or 16, but let’s multiply it out: (5 − 3) × (5 + 3) = (5 × 5) + (5 × 3) − (5 × 3) − (3 × ...