We Reason & We Prove for ALL Mathematics

Building Students' Critical Thinking, Grades 6-12

Fran Arbaugh, Margaret (Peg) S. Smith, Justin D. Boyle, Gabriel J. Stylianides, Michael D. Steele

• Publisher: Corwin
• Year: 2018
• ISBN:
  9781506378176
• Edition: 1
• Topic: Pedagogía
• Subtopic: Enseñanza de matemáticas

Sharpen concrete teaching strategies that empower students to reason-and-prove What does reasoning-and-proving instruction look like and how can teachers support students’ capacity to reason-and-prove? Designed as a learning tool for mathematics teachers in grades 6-12, this book transcends all mathematical content areas with a variety of activities for teachers that include

• Solving and discussing high-level mathematical tasks
• Analyzing narrative cases that make the relationship between teaching and learning salient
• Examining and interpreting student work
• Modifying curriculum materials and evaluating learning environments to better support students to reason-and-prove

No other book tackles reasoning-and-proving with such breath, depth, and practical applicability.

Chapter 1 Setting the Stage

Proof has historically been considered a topic first encountered in high school geometry class, featuring a two-column format of “statements” and “reasons.” In this chapter, we present reasoning-and-proving as a set of activities that transcends content and format and is accessible to all middle and high school students. Thinking about reasoning-and-proving more broadly will help you to enhance students’ understanding of the mathematics they are learning and their ability to construct valid mathematical arguments. While reading this chapter, we encourage you to consider

• how this broader perspective on reasoning-and-proving could benefit students’ understandings of mathematics; and
• what it would take to support students in building the capacity to engage in reasoning-and-proving.

Is Reasoning-and-Proving Really What You Think?

Over the past few years, the mathematics teachers at Hoover High School have been concerned about their students’ struggles to think and reason mathematically, which was made salient recently when they reviewed the results of an assessment that featured constructed response items. In general, they found that their students had difficulty explaining why an answer was correct beyond providing a procedural description (i.e., describing what they did). The teachers had also noticed that while the students were completing the assessment, they seemed to become quickly frustrated when faced with a task they could not easily and quickly solve. Many of the students’ responses were incomplete, and it looked like these students had just given up.

In an effort to improve this situation, all of the teachers in the math department committed to try to engage students in more tasks and activities that emphasize reasoning, justifying, and proving. While the students worked on these problems in small groups, the teachers also worked hard to ask more questions, listen to what students were saying, and to not do so much telling. It has not been easy!

Recently, the algebra teachers have been working on improving their students’ abilities to write proofs—not the formal two-column variety usually found in geometry—but rather algebraic, visual, and narrative arguments that can be used to explain “why things work” and to verify that something is true and it will work for all cases. In their latest professional learning community (PLC) meeting, they decided to give students the Sum of Three Consecutive Integers task (Figure 1.1). Their students had been working on exploring number theory tasks like this, so the teachers thought this would be a next task for them to do.

Figure 1.1 The Sum of Three Consecutive Integers task.

During the PLC meeting, teachers also agreed to identify things that happen during the lesson that they thought were “interesting” and to try to capture these events in some way (e.g., taking notes on a puzzling strategy, collecting samples of interesting student work, recording exchanges with students that they were troubled by). They also agreed to write short vignettes from these artifacts to share with each other. The teachers planned to discuss and analyze the vignettes below at their next PLC meeting.

Teaching Takeaway:

Collaborating with colleagues face-to-face or virtually provides an opportunity to share ideas, get feedback on your work, and, in general, support your efforts to improve instruction.

Vignette 1: Carly Epson’s Algebra Class

When I approached Shonda’s desk, I noticed that she had created a set of examples that supported the conjecture. She had even written “All of these sums are divisible by three because the sum of their digits is divisible by 3. So it is true because I can’t find one that doesn’t work.”

I knew immediately that Shonda’s string of examples didn’t prove the conjecture, but I didn’t want to say that. My hope was by asking the question “How do you know it will always work?” she would have to think twice about what she had done and see the limitations in her approach. But as you can see in the following exchange, it didn’t have that effect at all!

Ms. Epson: How do you know it will always work?

Shonda: It has so far and I can’t find one that doesn’t work.

Ms. Epson: But how can you be sure?

Shonda: I am sure.

She is right—she will never find one that doesn’t work, so I certainly didn’t want to encourage her to try. But not finding a counterexample doesn’t mean it will always work. I wasn’t sure what to do next to help her move beyond examples. I told her to keep thinking about how she could convince me. But when I checked back later, she had made no progress.

Vignette 2: Jason Steiner’s Algebra Class

When I stopped to check in on Keisha, I observed that she had written the following: “The sum of three consecutive numbers is A + B + C. You can’t tell if it is divisible by three or not. There’s not enough information.”

I decided to start asking her some questions that were intended to move her to a more useful way of representing the three numbers. Here is the gist of the exchange:

Mr. Steiner: So, do you think the statement is true or false?

Keisha:You can’t tell because you don’t have enough information.

Mr. Steiner:What information do you need?

Keisha: You need to know what one of the numbers is so you can tell what the others will be.

Mr. Steiner: But suppose that the first number is x. What would the next one be?

Keisha: y?

Mr. Steiner: How much bigger than x is the one that comes after x?

Keisha: 1 more.

Mr. Steiner: 1 more. So could you write it as x + 1?

Keisha: I guess so.

Mr. Steiner: Then what would the next biggest number be?

Keisha: x + 2?

Mr. Steiner: So can you add those three numbers together?

Keisha: What three numbers?

I was getting frustrated with Keisha and had no idea what to do next besides telling her what to do. I looked around and noticed that Charles had started on an algebraic solution that resembled the one that I was trying to help Keisha develop, so I suggested that Keisha and Charles share their solutions and decide which one they liked best. In the end, Keisha had a solution that looked like Charles’s, but I wondered what she understood about it.

Vignette 3: Barbara Law’s Algebra Class

One pair of students, Michael and Marissa, asked if they could go get some tiles. I had no idea what they wanted tiles for, but I told them to help themselves to one of the bins of square tiles. When I checked in with them later, they had arranged the blocks as shown below.

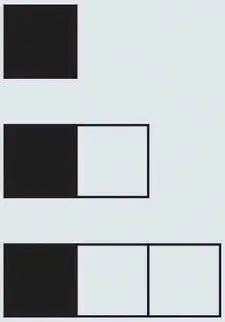
When I asked what the tiles represented, Michael explained, “If the black square is any number, then adding one square to it would be the next number and adding two squares to it would be the one after that.” Marissa continued, “If you add the three black squares together, you get three times the number and that is always divisible by three. Then, if you add the three white squares to that number, you will still get a number divisible by three because if you add three to a multiple of three, you get another multiple of three.”

I was caught off guard by this approach. I was looking for something more algebraic, and I wasn’t sure if this was correct or if it would really count as a proof. I told them it was “interesting” and suggested they try to use algebra to represent the tiles.

Vignette 4: Lynn Baker’s Algebra Class

I collected the work from students at the end of class and was not surprised to see that all of the students had clearly defined their variables (x = first number; x + 1 = second number; x + 2 = third number) because we did this as a class before they wrote their proofs. I was pleased that the students had gone on to show that x + x + 1 + x + 2 = 3x + 3. All of the students went on to say that 3x + 3 had to be divisible by 3 because, as Masey described when she was presenting her group’s work, “If you factor out a three, then the threes cancel.”

I was really pleased that they all were able to solve the problem and that they got the right answer. Clearly, my students are beginning to understand how to use algebra to prove things!

The work that these algebra teachers are engaged in to improve their students’ abilities to think deeply about mathematical concepts and relationships, reason mathematically, and write valid mathematical arguments is commendable. They came together in a PLC to work on their teaching and developed a common goal for improvement that was based on analyzing student data. They chose the same task to implement in their classrooms and gathered artifacts about interesting things that happened during class. They each came away from their class with questions to discuss with each other.

Carly Epson wondered what to do with a student who was convinced that a pattern would always hold true after only testing a few examples. Carly knew that testing examples was not sufficient for arguing the truth of a conjecture, but was unsure what to do next to support Shonda to move to a more generalized argument. Jason Steiner had a similar frustration with Keisha. Barbara Law’s student used a pictorial representation to make a generalized argument, and while it seemed convincing, Barbara wondered if this really counted as a proof of the conjecture. And while Lynn Baker felt that her students were well on their way to being able to write algebraic proofs, reading her vignette may have raised questions for you about what her students really knew and were able to do on their own, without her guidance with setting up the variables at the beginning of class.

Pause and Consider

What questions do these vignettes raise for you about mathematical reasoning-and-proving in the context of middle and high school mathematics?

Supporting Background and Contents of This Book

We designed this book to support you as you grapple with how to create learning environments that support middle and high school students to become deeper mathematical thinkers, better reasoners, and more capable of making sound mathematical arguments. We believe that this focus is the central work of mathematics education today and that students benefit when learning mathematics in these kind...