A Theory of Semiotics

Umberto Eco

• Publisher: Indiana University Press
• Year: 1976
• ISBN:
  9780253013316
• Topic: Literature
• Subtopic: Literary Criticism Theory

"... the greatest contribution to [semiotics] since the pioneering work of C. S. Peirce and Charles Morris." —Journal of Aesthetics and Art Criticism

"... draws on philosophy, linguistics, sociology, anthropology and aesthetics and refers to a wide range of scholarship... raises many fascinating questions." —Language in Society

"... a major contribution to the field of semiotic studies." —Robert Scholes, Journal of Aesthetics and Art Criticism

"... the most significant text on the subject published in the English language that I know of." —Arthur Asa Berger, Journal of Communication

Eco's treatment demonstrates his mastery of the field of semiotics. It focuses on the twin problems of the doctrine of signs—communication and signification—and offers a highly original theory of sign production, including a carefully wrought typology of signs and modes of production.

1: SIGNIFICATION
AND COMMUNICATION

1.1. An elementary communicational model

If every communication process must be explained as relating to a system of significations, it is necessary to single out the elementary structure of communication at the point where communication may be seen in its most elementary terms. Although every pattern of signification is a cultural convention, there is one communicative process in which there seems to be no cultural convention at all, but only – as was proposed in 0.7 – the passage of stimuli. This occurs when so-called physical ‘information’ is transmitted between two mechanical devices.

When a floating buoy signals to the control panel of an automobile the level reached by the gasoline, this process occurs entirely by means of a mechanical chain of causes and effects. Nevertheless, according to the principles of information theory, there is an ‘informational’ process that is in some way considered a communicational process too. Our example does not consider what happens once the signal (from the buoy) reaches the control panel and is converted into a visible measuring device (a red moving line or an oscillating arm): this is an undoubted case of sign-process in which the position of the arm stands for the level of the gasoline, in accordance with a conventionalized code.

But what is puzzling for a semiotic theory is the process which takes place before a human being looks at the pointer: although at the moment when he does so the pointer is the starting point of a signification process, before that moment it is only the final result of a preceding communicational process. During this process we cannot say that the position of the buoy stands for the movement of the pointer: instead of ‘standing-for’, the buoy stimulates, provokes, causes, gives rise to the movement of the pointer.

It is then necessary to gain a deeper knowledge of this type of process, which constitutes the lower threshold of semiotics. Let us outline a very simple communicative situation⁽¹⁾. An engineer – downstream – needs to know when a watershed located in a basin between two mountains, and closed by a Watergate, reaches a certain level of saturation, which he defines as ‘danger level’.

Whether there is water or not; whether it is above or below the danger level; how much above or below; at what rate it is rising: all this constitutes pieces of information which can be transmitted from the watershed, which will therefore be considered as a source of information.

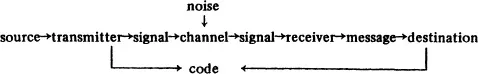
So the engineer puts in the watershed a sort of buoy which, when it reaches danger level, activates a transmitter capable of emitting an electric signal which travels through a channel (an electric wire) and is picked up downstream by a receiver, this device converts the signal into a given string of elements (i.e. releases a series of mechanical commands) that constitute a message for a destination apparatus. The destination, at this point, can release a mechanical response in order to correct the situation at the source (for instance opening the watergate so that the water can be slowly evacuated). Such a situation is usually represented as follows:

In this model the code is the device which assures that a given electric signal produces a given mechanical message, and that this elicits a given response. The engineer can establish the following code: presence of signal (+ A) versus absence of signal (- A). The signal + A is released when the buoy sensitizes the transmitter.

But this Watergate Model’ also foresees the presence of potential noise on the channel, which is to say any disturbance that could alter the nature of the signals, making them difficult to detect, or producing + A when - A is intended and vice versa. Therefore the engineer has to complicate his code. For instance, if he establishes two different levels of signal, namely + A and +B, he then disposes of three signals ⁽²⁾ and the destination may accordingly be instructed in order to release three kinds of response.

+ A produces ‘state of rest’

+ B produces ‘feedback’

- AB (and + AB) produces an emergency signal (meaning that something does not work)

This complication of the code increases the cost of the entire apparatus but makes the transmission of information more secure. Nevertheless there can be so much noise as to produce + A instead of + B. In order to avoid this risk, the code must be considerably complicated. Suppose that the engineer now disposes of four positive signals and establishes that every message must be composed of two signals. The four positive signals can be represented by four different levels but in order to better control the entire process the engineer decides to represent them by four electric bulbs as well. They can be set out in a positional series, so that A is recognizable inasmuch as it precedes B and so on; they can also be designed as four bulbs of differing colors, following a wave-length progression (green, yellow, orange, red). It must be made absolutely clear that the destination apparatus does not need to ‘see’ bulbs (for it has no sensory organs): but the bulbs are useful for the engineer so that he can follow what is happening.

I should add that the correspondence between electric signals (received by the transmitter and translated into mechanical messages) and the lighting of the bulbs (obviously activated by another receiver) undoubtedly constitutes a new coding phenomenon that would need to receive separate attention; but for the sake of convenience I shall consider both the message to the destination and the bulbs as two aspects of the same phenomenon. At this point the engineer has – at least from a theoretical point of view – 16 possible messages at his disposal:

AA BA CA DA

AB BB CB DB

AC BC CC DC

AD BD CD DD

Since AA, BB, CC, DD are simply repetitions of a single signal, and therefore cannot be instantaneously emitted, and since six messages are simply the reverse of six others (for instance, BA is the reverse of AB, and the temporal succession of two signals is not being considered in this case), the engineer actually disposes of six messages: AB, BC, CD, AD, AC and BD. Suppose that he assigns to the message AB the task of signalling “danger level”. He has at his disposal 5 ‘empty’ messages.

Thus the engineer has achieved two interesting results: (i) it is highly improbable that a noise will activate two wrong bulbs and it is probable that any wrong activation will give rise to a ‘senseless’ message, such as ABC or ABCD: therefore it is easier to detect a misfunctioning; (ii) since the code has been complicated and the cost of the transmission has been increased, the engineer may take advantage of this investment to amortize it through a more informative exploitation of the code.

In fact with such a code he can get a more comprehensive range of information about what happens at the source and he can better instruct the destination, selecting more events to be informed about and more mechanical responses to be released by the apparatus in order to control the entire process more tightly. He therefore establishes a new code, able to signal more states of the water in the watershed and to elicit more articulated responses (Table 4).

Table 4

(a)		(b)		(c)
bulbs		states of water		responses of the
		or		destination
		notions about the
		states of water
AB	=	danger level	=	water dumping
BC	=	alarm level	=	state of alarm
CD	=	security level	=	state of rest
AD	=	insufficency level	=	water make-up

The fact of having complicated the code has introduced redundancy into it: two signals are used in order to give one piece of information. But the redundancy has also provided a supply of messages, thus enabling the engineer to recognize a larger array of situations at the source and to establish a larger array of responses at the destination. As a matter of fact redundancy has also provided two more messages (AC and BD) that the engineer does not want to use and by means of which he could signal other states within the watershed (combined with appropriate additional responses): they could also be used in order to introduce synonymies (danger level being signalled both by AB and by AC). Anyway the code which has been adopted would seem to be an optimal one for an engineer’s purposes and it would be unwise to complicate it too much.⁽³⁾

1.2. Systems and codes

Once the Watergate Model is established and the engineer has finished his project, a semiotician could ask him a few questions, such as: (i) what do you call a ‘code’? the device by which you know that a given state in the watershed corresponds to a given set of illuminated bulbs? (ii) if so, does the mechanical apparatus possess a code, that is, does the destination recognize the ‘meaning’ of the received message or does it simply respond to mechanical stimuli? (iii) and is the fact that the destination responds to a given array of stimuli by means of a given sequence of responses based on a code? (iv) who is that code for? you or the apparatus? (v) and anyway, is it not true that many people would call the internal organization of the system of bulbs a code, irrespective of the state of things that can be signalled through its combinational articultation? (vi) finally, is not the fact that the water’s infinite number of potential positions within the watershed have been segmented into four, and only four ‘pertinent’ states, sometimes called a ‘code’?

One could carry on like this for a long time. But it seems unnecessary, since it will already be quite clear that under the name of /code/ the engineer is considering at least four different phenomena:

(a) A set of signals ruled by internal combinatory laws These signals are not necessarily connected or connectable with the state of the water that they conveyed in the Watergate Model, nor with the destination responses that the engineer decided they should be allowed to elicit. They could convey different notions about things and they could elicit a different set of responses: for instance they could be used to communicate the engineer’s love for the next-watershed girl, or to persuade the girl to return his passion. Moreover these signals can travel through the channel without conveying or eliciting anything, simply in order to test the mechanical efficiency of the transmitting and receiving apparatuses. Finally they can be considered as a pure combinational structure that only takes the form of electric signals by chance, an interplay of empty positions and mutual oppositions, as will be seen in 1.3. They could be called a syntactic system.

(b) A set of states of the water which are taken into account as a set of notions about the state of the water and which can become (as happened in the Watergate Model) a set of possible co...