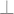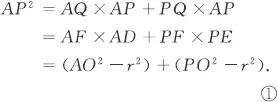Mathematical Olympiad In China (2009-2010): Problems And Solutions

Problems and Solutions

Bin Xiong, Peng Yee Lee

• Publisher: WSPC
• Year: 2013
• ISBN:
  9789814397438
• Topic: Mathematik
• Subtopic: Mathematik Allgemein

The International Mathematical Olympiad (IMO) is a competition for high school students. China has taken part in the IMO 21 times since 1985 and has won the top ranking for countries 14 times, with a multitude of golds for individual students. The six students China has sent every year were selected from 20 to 30 students among approximately 130 students who took part in the annual China Mathematical Competition during the winter months. This volume of comprises a collection of original problems with solutions that China used to train their Olympiad team in the years from 2009 to 2010. Mathematical Olympiad problems with solutions for the years 2002–2008 appear in an earlier volume, Mathematical Olympiad in China.

Contents:

• 2008–2009 China Mathematical Competition
• 2008–2009 China Mathematical Competition (Complementary Test)
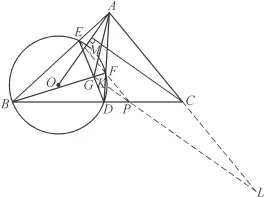
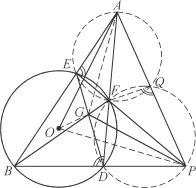
• 2009–2010 China Mathematical Olympiad
• 2009–2010 China National Team Selection Test
• 2008–2009 China Girls' Mathematical Olympiad
• 2008–2009 China Western Mathematical Olympiad
• 2009–2010 China Southeastern Mathematical Olympiad
• 2009–2010 International Mathematical Olympiad

Readership: Mathematics students, school teachers, college lecturers, university professors; mathematics enthusiasts.

China National
Team Selection Test

2009 (Wuhan, Hubei)

First Day

(0800 – 1230; March 31, 2009)

Let D be a point on side BC of triangle ABC such that

CAD =

CBA. A circle with center O passes through B, D, and meets segments AB, AD at E, F, respectively. Lines BF and DE meet at point G. M is the midpoint of AG. Prove that CM

AO. (Posed by Xiong Bin)

Proof As shown in Fig. 1, extend EF and meet BC at point P, and join and extend GP, which meets AD at K and the extension of AC at L.

Fig. 1

As shown in Fig. 2, let Q be a point on AP such that

PQF =

AEF =

ADB.

It is easy to see that A, E, F, Q and F, D, P, Q are concyclic respectively. Denote by r the radius of

O. By the power of a point theorem,

Fig. 2

Similarly,

By

,

, we have AP² − AG² = PO² − GO², which implies that PG

AO. As shown in Fig. 3, applying Menelaus’ theorem for ΔPFD and line AEB, we have

Applying Ceva's theorem for ΔPFD and point G, we have

yields

Fig. 3

Equation

illustrates that A, K are harmonic points with respect to F, D, i.e. AF × KD = AD × FK.

It follows that

...