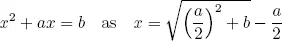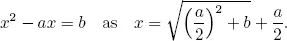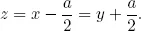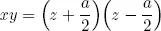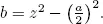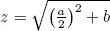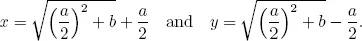Galois' Theory of Algebraic Equations

Jean-Pierre Tignol

• Éditeur: WSPC
• Année: 2015
• ISBN:
  9789814704717
• Édition: 2
• Sujet: Mathématiques
• Sous-sujet: Mathématiques générales

The book gives a detailed account of the development of the theory of algebraic equations, from its origins in ancient times to its completion by Galois in the nineteenth century. The appropriate parts of works by Cardano, Lagrange, Vandermonde, Gauss, Abel, and Galois are reviewed and placed in their historical perspective, with the aim of conveying to the reader a sense of the way in which the theory of algebraic equations has evolved and has led to such basic mathematical notions as "group" and "field".

A brief discussion of the fundamental theorems of modern Galois theory and complete proofs of the quoted results are provided, and the material is organized in such a way that the more technical details can be skipped by readers who are interested primarily in a broad survey of the theory.

In this second edition, the exposition has been improved throughout and the chapter on Galois has been entirely rewritten to better reflect Galois' highly innovative contributions. The text now follows more closely Galois' memoir, resorting as sparsely as possible to anachronistic modern notions such as field extensions. The emerging picture is a surprisingly elementary approach to the solvability of equations by radicals, and yet is unexpectedly close to some of the most recent methods of Galois theory.

Request Inspection Copy

The book gives a detailed account of the development of the theory of algebraic equations, from its origins in ancient times to its completion by Galois in the nineteenth century. The appropriate parts of works by Cardano, Lagrange, Vandermonde, Gauss, Abel, and Galois are reviewed and placed in their historical perspective, with the aim of conveying to the reader a sense of the way in which the theory of algebraic equations has evolved and has led to such basic mathematical notions as "group" and "field".

A brief discussion of the fundamental theorems of modern Galois theory and complete proofs of the quoted results are provided, and the material is organized in such a way that the more technical details can be skipped by readers who are interested primarily in a broad survey of the theory.

In this second edition, the exposition has been improved throughout and the chapter on Galois has been entirely rewritten to better reflect Galois' highly innovative contributions. The text now follows more closely Galois' memoir, resorting as sparsely as possible to anachronistic modern notions such as field extensions. The emerging picture is a surprisingly elementary approach to the solvability of equations by radicals, and yet is unexpectedly close to some of the most recent methods of Galois theory.

Request Inspection Copy

Readership: Upper level undergraduates, graduate students and mathematicians in algebra.
Key Features:

• Describes the problems and methods at the origin of modern abstract algebra
• Provides an elementary thorough discussion of the insolvability of general equations of degree at least five and of ruler-and-compass constructions
• Original exposition relying on early sources to set classical Galois theory into its historical perspective

Chapter 1

Quadratic Equations

Since the solution of a linear equation ax = b does not use anything more than a division, it hardly belongs to the algebraic theory of equations; it is therefore appropriate to begin our discussion with quadratic equations

ax² + bx + c = 0(a ≠ 0).

Dividing each side by a, we reduce to the case where the coefficient of x² is 1:

x² + px + q = 0.

The solution of this equation is well-known: when

is added to each side, the square of

appears and the equation can be written

(This procedure is called “completion of the square.”) The values of x easily follow:

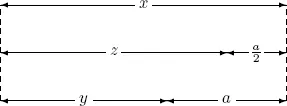
This formula is so well-known that it may be rather surprising to note that the solution of quadratic equations could not have been written in this form before the seventeenth century.¹ Nevertheless, mathematicians had been solving quadratic equations for about 40 centuries before. The purpose of this first chapter is to give a brief outline of this “prehistory” of the theory of quadratic equations.

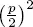
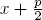
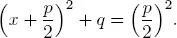
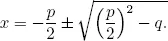
1.1Babylonian algebra

The first known solution of a quadratic equation dates from about 2000 B.C.; on a Babylonian tablet, one reads (see van der Waerden [79, p. 69])

I have subtracted from the area the side of my square: 14.30. Take 1, the coefficient. Divide 1 into two parts: 30. Multiply 30 and 30: 15. You add to 14.30, and 14.30.15 has the root 29.30. You add to 29.30 the 30 which you have multiplied by itself: 30, and this is the side of the square.

This text obviously provides a procedure for finding the side of a square (say x) when the difference between the area and the side (i.e., x² − x) is given; in other words, it gives the solution of x² − x = b.

However, one may be puzzled by the strange arithmetic used by Babylonians. It can be explained by the fact that their base for numeration is 60; therefore 14.30 really means 14 · 60 + 30, i.e., 870. Moreover, they had no symbol to indicate the absence of a number or to indicate that certain numbers are intended as fractions. For instance, when 1 is divided by 2, the result which is indicated as 30 really means 30 · 60⁻¹, i.e., 0.5. The square of this 30 is then 15 which means 0.25, and this explains why the sum of 14.30 and 15 is written as 14.30.15: in modern notation, the operation is 870 + 0.25 = 870.25.

After clearing the notational ambiguities, it appears that the author correctly solves the equation x² − x = 870, and gets x = 30. The other solution x = −29 is neglected, since the Babylonians had no negative numbers.

This lack of negative numbers prompted Babylonians to consider various types of quadratic equations, depending on the signs of coefficients. There are three types in all:

x² + ax = b,x² − ax = b,andx² + b = ax,

where a, b stand for positive numbers. (The fourth type x² + ax + b = 0 obviously has no (positive) solution.)

Babylonians could not have written these various types in this form, since they did not use letters in place of numbers, but from the example above and from other numerical examples contained on the same tablet, it clearly appears that the Babylonians knew the solution of

and of

How they argued to get these solutions is not known, since in every extant example, only the procedure to find the solution is described, as in the example above. It is very likely that they had previously found the solution of geometric problems, such as to find the length and the breadth of a rectangle, when the excess of the length on the breadth and the area are given. Letting x and y respectively denote the length and the breadth of the rectangle, this problem amounts to solving the system

By elimination of y, this system yields the following equation for x:

If x is eliminated instead of y, we get

Conversely, equations (1.2) and (1.3) are equivalent to system (1.1) after setting y = x − a or x = y + a.

They probably deduced their solution for quadratic equations (1.2) and (1.3) from their solution of the corresponding system (1.1), which could be obtained as follows: let z be the arithmetic mean of x and y.

In other words, z is the side of the square which has the same perimeter as the given rectangle:

Compare then the area of the square (i.e., z²) to the area of the rectangle (xy = b). We have

hence

Therefore,

and it follows that

This solves at once the quadratic equations x² − ax = b and y² + ay = b.

Looking at the various examples of quadratic equations solved by Babylonians, one notices a curious fact: the third type x² + b = ax does not explicitly appear. This is even more puzzling in view of the frequent occurrence in Babylonian tablets of problems such as to find the length and the breadth of a rectangle when the perimeter and the area of the rectangle are given, which amounts to the solution of

By elimination of y, this system leads to x² + b = ax. So, why did Babylonians solve...